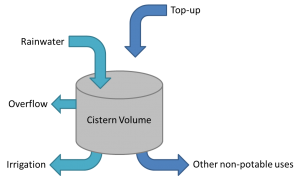
Difference between revisions of "Rainwater harvesting: Sizing and modeling"
Jenny Hill (talk | contribs) |
Jenny Hill (talk | contribs) m (→Simple) |
||
| Line 3: | Line 3: | ||
===Simple=== | ===Simple=== | ||
Five percent of the average annual yield can be estimated: | Five percent of the average annual yield can be estimated: | ||
| − | + | <math>Y_{0.05} = A_{c} \times C_{vol,A}\times R_{a} \times e \times 0.05</math> | |
| − | + | {{plainlist|Where: | |
| − | {{plainlist| | + | *''Y<sub>0.05</sub>'' is five percent of the average annual yield (L) |
| − | *''Y<sub>0.05</sub>'' | + | *''A<sub>c</sub>'' is the catchment area (m<sup>2</sup>) |
| − | *''A'' | + | *''C<sub>vol, A</sub>'' is the annual runoff coefficient for the catchment |
| − | *''C<sub>vol, A</sub>'' | + | *''R<sub>a</sub>'' is the average annual rainfall depth (mm) |
| − | *''R<sub>a</sub>'' | + | *''e'' is the efficiency of the pre-storage filter}} |
| − | *''e'' | ||
*Filter efficiency (''e'') can be reasonably estimated as 0.9 pending manufacturer’s information.<br> | *Filter efficiency (''e'') can be reasonably estimated as 0.9 pending manufacturer’s information.<br> | ||
*In a study of three sites in Ontario, STEP found the annual ''C<sub>vol, A</sub>'' of the rooftops to be around 0.8 [http://www.sustainabletechnologies.ca/wp/home/urban-runoff-green-infrastructure/low-impact-development/rainwater-harvesting/performance-evaluation-of-rainwater-harvesting-systems-toronto-ontario/]. This figure includes losses to evaporation, snow being blown off the roof, and a number of overflow events. | *In a study of three sites in Ontario, STEP found the annual ''C<sub>vol, A</sub>'' of the rooftops to be around 0.8 [http://www.sustainabletechnologies.ca/wp/home/urban-runoff-green-infrastructure/low-impact-development/rainwater-harvesting/performance-evaluation-of-rainwater-harvesting-systems-toronto-ontario/]. This figure includes losses to evaporation, snow being blown off the roof, and a number of overflow events. | ||
| − | |||
Five percent of the average annual demand can be estimated: | Five percent of the average annual demand can be estimated: | ||
| − | + | <math>D_{0.05} = P_{d} \times #\times 18.25</math> | |
| − | + | {{plainlist|Where: | |
| − | {{plainlist| | + | *''D<sub>0.05</sub>'' is five percent of the average annual demand (L) |
| − | *''D<sub>0.05</sub>'' | + | *''P<sub>d</sub>'' is the daily demand per person (L) |
| − | *''P<sub>d</sub>'' | + | *''#'' is the number of occupants}} |
| − | *'' | ||
| − | |||
Then the following calculations are based upon two criteria: | Then the following calculations are based upon two criteria: | ||
| Line 30: | Line 26: | ||
When \(Y_{0.05}/D_{0.05}<0.33\), the storage volume required can be estimated: | When \(Y_{0.05}/D_{0.05}<0.33\), the storage volume required can be estimated: | ||
<math>V_{S} = A \times C_{vol,E}\times R_{d} \times e</math> | <math>V_{S} = A \times C_{vol,E}\times R_{d} \times e</math> | ||
| − | + | {{plainlist|Where: | |
| − | {{plainlist| | ||
*''V<sub>S</sub>'' = Storage volume required (L) | *''V<sub>S</sub>'' = Storage volume required (L) | ||
*''A'' = The catchment area (m<sup>2</sup>) | *''A'' = The catchment area (m<sup>2</sup>) | ||
Revision as of 18:56, 20 February 2018
Simple[edit]
Five percent of the average annual yield can be estimated:
Where:
- Y0.05 is five percent of the average annual yield (L)
- Ac is the catchment area (m2)
- Cvol, A is the annual runoff coefficient for the catchment
- Ra is the average annual rainfall depth (mm)
- e is the efficiency of the pre-storage filter
- Filter efficiency (e) can be reasonably estimated as 0.9 pending manufacturer’s information.
- In a study of three sites in Ontario, STEP found the annual Cvol, A of the rooftops to be around 0.8 [1]. This figure includes losses to evaporation, snow being blown off the roof, and a number of overflow events.
Five percent of the average annual demand can be estimated: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D_{0.05} = P_{d} \times #\times 18.25}
Where:
- D0.05 is five percent of the average annual demand (L)
- Pd is the daily demand per person (L)
- # is the number of occupants
Then the following calculations are based upon two criteria:
- A design rainfall depth is to be captured entirely by the RWH system.
- The average annual demand (D) is greater than the average annual yield (Y) from the catchment.
When \(Y_{0.05}/D_{0.05}<0.33\), the storage volume required can be estimated:
Where:
- VS = Storage volume required (L)
- A = The catchment area (m2)
- Cvol,E = The design storm runoff coefficient for the catchment
- Rd = The design storm rainfall depth (mm), and
- e = The efficiency of the pre-storage filter.
- Careful catchment selection means that the runoff coefficient, for a rainstorm event (Cvol, E) should be 0.9 or greater.
Finally, when \(0.33<Y_{0.05}/D_{0.05}<0.7\), the total storage required can be estimated by adding Y0.05:
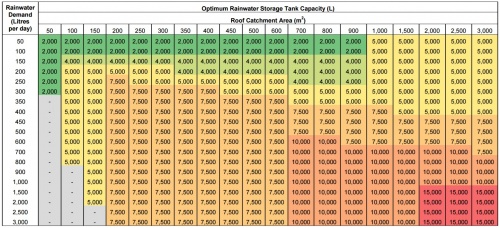
STEP Rainwater Harvesting Tool[edit]
The Sustainable Technologies Evaluation Program have produced a rainwater harvesting design and costing tool specific to Ontario. The tool is in a simple to use Excel format and is free to download.